… with laughter.
Without further ado, here is what transpired moments ago.
vttoth: Can you produce TikZ code that draws a cat?
ChatGPT: Drawing a cat using TikZ can be quite complex, but here’s a simple representation of a cat using TikZ:
\documentclass[tikz, border=2mm]{standalone}
\begin{document}
\begin{tikzpicture}
% Body
\draw[fill=gray!30] (0,0) ellipse (1cm and 1.5cm);
% Head
\draw[fill=gray!30] (0,1.75) circle (0.75cm);
% Eyes
\fill[white] (-0.35,2.1) circle (0.25cm);
\fill[white] (0.35,2.1) circle (0.25cm);
\fill[black] (-0.35,2.1) circle (0.1cm);
\fill[black] (0.35,2.1) circle (0.1cm);
% Nose and mouth
\fill[pink] (0,1.8) circle (0.1cm);
\draw (0,1.8) -- (0,1.6);
\draw (0,1.6) -- ++(-0.15,-0.15);
\draw (0,1.6) -- ++(0.15,-0.15);
% Ears
\draw[fill=gray!30] (-0.75,2.5) -- (-0.4,2.4) -- (-0.65,2.0) -- cycle;
\draw[fill=gray!30] (0.75,2.5) -- (0.4,2.4) -- (0.65,2.0) -- cycle;
% Tail
\draw[thick, gray!30] (1.3,-0.5) to[out=-90,in=0] (0.5,-1.5) to[out=-180,in=-90] (-0.5,-0.5);
\end{tikzpicture}
\end{document}
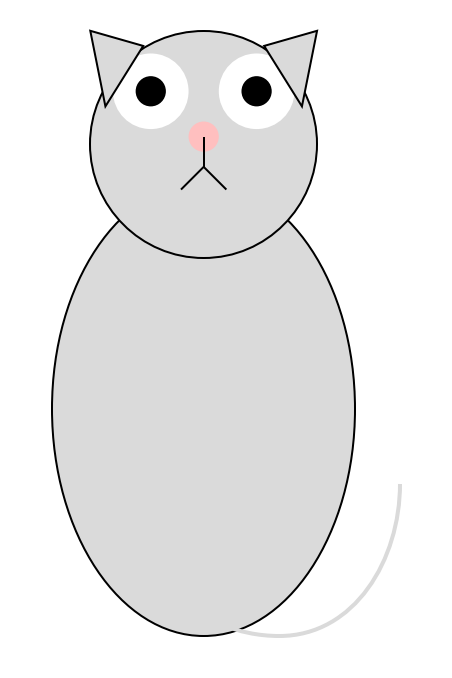
This code will produce a simple cat illustration. Copy and paste the code into a LaTeX editor that supports the TikZ
package, or use an online LaTeX renderer like Overleaf to generate the cat drawing. Please note that this is a simplistic
and stylized representation of a cat and not a realistic drawing.
vttoth: I love it. I find the result irresistibly funny. Is it a copy of a TikZ example that you came across during your
training or did you come up with this image on your own?
ChatGPT: I’m glad you liked the cat drawing! The cat illustration was not copied from a specific TikZ example that
I came across during my training. Instead, I synthesized it on my own, using my understanding of TikZ commands and
my knowledge of cat features to create a simple and stylized representation of a cat.
vttoth: Can you add whiskers?
ChatGPT: Of course! Here’s the updated TikZ code for the cat drawing with whiskers added:
\documentclass[tikz, border=2mm]{standalone}
\begin{document}
\begin{tikzpicture}
% Body
\draw[fill=gray!30] (0,0) ellipse (1cm and 1.5cm);
% Head
\draw[fill=gray!30] (0,1.75) circle (0.75cm);
% Eyes
\fill[white] (-0.35,2.1) circle (0.25cm);
\fill[white] (0.35,2.1) circle (0.25cm);
\fill[black] (-0.35,2.1) circle (0.1cm);
\fill[black] (0.35,2.1) circle (0.1cm);
% Nose and mouth
\fill[pink] (0,1.8) circle (0.1cm);
\draw (0,1.8) -- (0,1.6);
\draw (0,1.6) -- ++(-0.15,-0.15);
\draw (0,1.6) -- ++(0.15,-0.15);
% Ears
\draw[fill=gray!30] (-0.75,2.5) -- (-0.4,2.4) -- (-0.65,2.0) -- cycle;
\draw[fill=gray!30] (0.75,2.5) -- (0.4,2.4) -- (0.65,2.0) -- cycle;
% Whiskers
\foreach \i in {-1, 1} {
\draw (\i*0.15,1.65) -- (\i*0.5,1.5);
\draw (\i*0.15,1.65) -- (\i*0.5,1.65);
\draw (\i*0.15,1.65) -- (\i*0.5,1.8);
}
% Tail
\draw[thick, gray!30] (1.3,-0.5) to[out=-90,in=0] (0.5,-1.5) to[out=-180,in=-90] (-0.5,-0.5);
\end{tikzpicture}
\end{document}
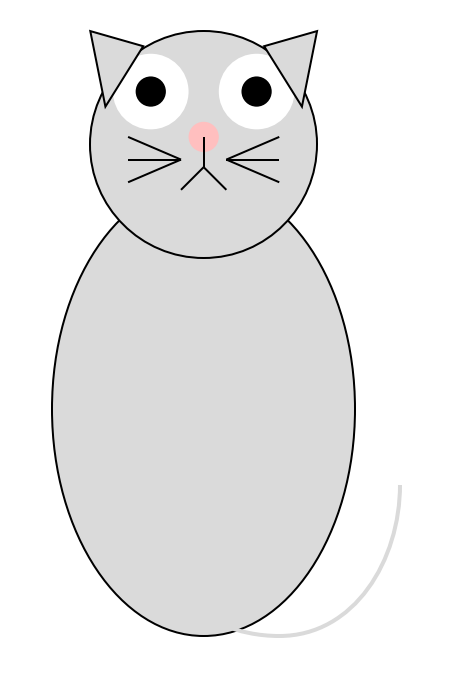
This code includes the addition of whiskers to the cat’s face. Copy and paste the code into a LaTeX editor that
supports the TikZ package or use an online LaTeX renderer like Overleaf to generate the updated cat drawing with
whiskers.
I am laughing my head off in part because these cats, especially now with the whiskers added, strongly resemble cats I used to draw on letter-size sheets of paper with my set of colored pencils when I was of kindergarten age.
But I am also staring at these images sensing a combination of awe and apprehension.